The ability to do work and what waves transfer

16 Waves

16.4 Energy and Power of a Wave

Learning Objectives

Past the end of this section, you will exist able to:

Explain how energy travels with a pulse or wave
Describe, using a mathematical expression, how the free energy in a wave depends on the amplitude of the wave

All waves behave energy, and sometimes this can exist straight observed. Earthquakes can shake whole cities to the ground, performing the work of thousands of wrecking assurance ((Figure)). Loud sounds can pulverize nerve cells in the inner ear, causing permanent hearing loss. Ultrasound is used for deep-heat treatment of muscle strains. A laser beam can burn away a malignancy. Water waves chew up beaches.

Property damaged by an earthquake. Many buildings have collapsed. — **Figure sixteen.fifteen** The destructive upshot of an earthquake is observable evidence of the free energy carried in these waves. The Richter calibration rating of earthquakes is a logarithmic scale related to both their amplitude and the free energy they carry.

In this department, we examine the quantitative expression of free energy in waves. This volition exist of central importance in later discussions of waves, from sound to lite to quantum mechanics.

Energy in Waves

The amount of energy in a wave is related to its aamplitude and its frequency. Big-aamplitude earthquakes produce big basis displacements. Loud sounds take high-pressure amplitudes and come from larger-aamplitude source vibrations than soft sounds. Big ocean breakers churn upwards the shore more than small ones. Consider the example of the seagull and the h2o moving ridge before in the chapter ((Figure)). Work is done on the seagull past the wave as the seagull is moved up, changing its potential free energy. The larger the amplitude, the higher the seagull is lifted past the moving ridge and the larger the modify in potential energy.

The energy of the moving ridge depends on both the amplitude and the frequency. If the energy of each wavelength is considered to be a detached package of energy, a high-frequency wave volition deliver more of these packets per unit time than a low-frequency wave. We will see that the average rate of free energy transfer in mechanical waves is proportional to both the foursquare of the amplitude and the square of the frequency. If two mechanical waves have equal amplitudes, but one wave has a frequency equal to twice the frequency of the other, the higher-frequency moving ridge will take a rate of energy transfer a factor of 4 times as great as the rate of energy transfer of the lower-frequency wave. It should be noted that although the rate of energy transport is proportional to both the square of the aamplitude and square of the frequency in mechanical waves, the rate of free energy transfer in electromagnetic waves is proportional to the square of the amplitude, but contained of the frequency.

Ability in Waves

Consider a sinusoidal moving ridge on a cord that is produced past a string vibrator, as shown in (Figure). The cord vibrator is a device that vibrates a rod up and downwardly. A string of uniform linear mass density is fastened to the rod, and the rod oscillates the string, producing a sinusoidal moving ridge. The rod does work on the string, producing energy that propagates along the string. Consider a mass chemical element of the string with a mass

$\text{Δ}m$

, as seen in (Figure). As the free energy propagates forth the string, each mass element of the string is driven upwards and downwardly at the aforementioned frequency as the wave. Each mass element of the string can be modeled as a elementary harmonic oscillator. Since the string has a constant linear density

$\mu =\frac{\text{Δ}m}{\text{Δ}x},$

each mass element of the string has the mass

$\text{Δ}m=\mu \text{Δ}x.$

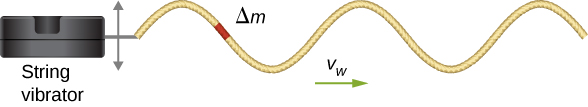
Figure shows a box to the left, labeled string vibrator. A string is attached to this and forms a transverse wave that propagates towards the right with velocity v subscript w. A small portion of the string is highlighted and is labeled delta m. — **Figure 16.xvi** A string vibrator is a device that vibrates a rod. A string is attached to the rod, and the rod does work on the string, driving the string upward and down. This produces a sinusoidal wave in the string, which moves with a wave velocity v. The moving ridge speed depends on the tension in the string and the linear mass density of the string. A section of the string with mass
$\text{Δ}m$

oscillates at the same frequency every bit the moving ridge.

The total mechanical energy of the wave is the sum of its kinetic free energy and potential energy. The kinetic free energy

$K=\frac{1}{2}m{v}^{2}$

of each mass element of the string of length

$\text{Δ}x$

$\text{Δ}K=\frac{1}{2}(\text{Δ}m){v}_{y}^{2},$

equally the mass element oscillates perpendicular to the direction of the motion of the wave. Using the abiding linear mass density, the kinetic energy of each mass chemical element of the string with length

$\text{Δ}x$

$\text{Δ}K=\frac{1}{2}(\mu \text{Δ}x){v}_{y}^{2}.$

A differential equation tin can be formed past letting the length of the mass element of the string approach naught,

$dK=\underset{\text{Δ}x\to 0}{\text{lim}}\frac{1}{2}(\mu \text{Δ}x){v}_{y}^{2}=\frac{1}{2}(\mu dx){v}_{y}^{2}.$

Since the wave is a sinusoidal moving ridge with an angular frequency

$\omega ,$

the position of each mass element may be modeled every bit

$y(x,t)=A\,\text{sin}(kx-\omega t).$

Each mass element of the string oscillates with a velocity

${v}_{y}=\frac{\partial y(x,t)}{\partial t}=\text{−}A\omega \,\text{cos}(kx-\omega t).$

The kinetic energy of each mass element of the string becomes

$\begin{array}{cc}\hfill dK& =\frac{1}{2}(\mu dx){(\text{−}A\omega \,\text{cos}(kx-\omega t))}^{2},\hfill \\ & =\frac{1}{2}(\mu dx){A}^{2}{\omega }^{2}\,{\text{cos}}^{2}(kx-\omega t).\hfill \end{array}$

The wave can be very long, consisting of many wavelengths. To standardize the energy, consider the kinetic free energy associated with a wavelength of the wave. This kinetic energy can be integrated over the wavelength to find the energy associated with each wavelength of the wave:

$\begin{array}{ccc}\hfill dK& =\hfill & \frac{1}{2}(\mu dx){A}^{2}{\omega }^{2}\,{\text{cos}}^{2}(kx),\hfill \\ \hfill \underset{0}{\overset{{K}_{\lambda }}{\int }}dK& =\hfill & \underset{0}{\overset{\lambda }{\int }}\frac{1}{2}\mu {A}^{2}{\omega }^{2}\,{\text{cos}}^{2}(kx)dx=\frac{1}{2}\mu {A}^{2}{\omega }^{2}\underset{0}{\overset{\lambda }{\int }}{\text{cos}}^{2}(kx)dx,\hfill \\ \hfill {K}_{\lambda }& =\hfill & \frac{1}{2}\mu {A}^{2}{\omega }^{2}{[\frac{1}{2}x+\frac{1}{4k}\text{sin}(2kx)]}_{0}^{\lambda }=\frac{1}{2}\mu {A}^{2}{\omega }^{2}[\frac{1}{2}\lambda +\frac{1}{4k}\text{sin}(2k\lambda )-\frac{1}{4k}\text{sin}(0)],\hfill \\ \hfill {K}_{\lambda }& =\hfill & \frac{1}{4}\mu {A}^{2}{\omega }^{2}\lambda .\hfill \end{array}$

There is also potential free energy associated with the wave. Much like the mass oscillating on a spring, there is a conservative restoring force that, when the mass element is displaced from the equilibrium position, drives the mass element back to the equilibrium position. The potential energy of the mass element tin be found by because the linear restoring force of the string, In Oscillations, we saw that the potential free energy stored in a spring with a linear restoring force is equal to

$U=\frac{1}{2}{k}_{s}{x}^{2},$

where the equilibrium position is divers every bit

$x=0.00\,\text{m}.$

When a mass attached to the spring oscillates in unproblematic harmonic motility, the angular frequency is equal to

$\omega =\sqrt{\frac{{k}_{s}}{m}}.$

As each mass element oscillates in unproblematic harmonic motility, the spring constant is equal to

${k}_{s}=\text{Δ}m{\omega }^{2}.$

The potential energy of the mass chemical element is equal to

$\text{Δ}U=\frac{1}{2}{k}_{s}{x}^{2}=\frac{1}{2}\text{Δ}m{\omega }^{2}{x}^{2}.$

Note that

${k}_{s}$

is the spring constant and not the wave number

$k=\frac{2\pi }{\lambda }.$

This equation tin can be used to discover the energy over a wavelength. Integrating over the wavelength, we can compute the potential free energy over a wavelength:

$\begin{array}{ccc}\hfill dU& =\hfill & \frac{1}{2}{k}_{s}{x}^{2}=\frac{1}{2}\mu {\omega }^{2}{x}^{2}dx,\hfill \\ \hfill {U}_{\lambda }& =\hfill & \frac{1}{2}\mu {\omega }^{2}{A}^{2}\underset{0}{\overset{\lambda }{\int }}{\text{cos}}^{2}(kx)dx=\frac{1}{4}\mu {A}^{2}{\omega }^{2}\lambda .\hfill \end{array}$

The potential free energy associated with a wavelength of the moving ridge is equal to the kinetic free energy associated with a wavelength.

The total energy associated with a wavelength is the sum of the potential energy and the kinetic energy:

$\begin{array}{c}{E}_{\lambda }={U}_{\lambda }+{K}_{\lambda },\hfill \\ {E}_{\lambda }=\frac{1}{4}\mu {A}^{2}{\omega }^{2}\lambda +\frac{1}{4}\mu {A}^{2}{\omega }^{2}\lambda =\frac{1}{2}\mu {A}^{2}{\omega }^{2}\lambda .\hfill \end{array}$

The time-averaged power of a sinusoidal mechanical wave, which is the boilerplate rate of free energy transfer associated with a wave as it passes a indicate, tin exist establish by taking the full energy associated with the wave divided by the fourth dimension it takes to transfer the energy. If the velocity of the sinusoidal wave is constant, the time for ane wavelength to pass by a betoken is equal to the menstruum of the wave, which is likewise constant. For a sinusoidal mechanical wave, the fourth dimension-averaged power is therefore the energy associated with a wavelength divided past the period of the moving ridge. The wavelength of the wave divided by the period is equal to the velocity of the wave,

${P}_{\text{ave}}=\frac{{E}_{\lambda }}{T}=\frac{1}{2}\mu {A}^{2}{\omega }^{2}\frac{\lambda }{T}=\frac{1}{2}\mu {A}^{2}{\omega }^{2}v.$

Note that this equation for the time-averaged power of a sinusoidal mechanical wave shows that the ability is proportional to the foursquare of the aamplitude of the wave and to the square of the angular frequency of the wave. Call up that the angular frequency is equal to

$\omega =2\pi f$

, so the power of a mechanical wave is equal to the square of the amplitude and the square of the frequency of the wave.

Example

Power Supplied by a String Vibrator

Consider a two-meter-long string with a mass of 70.00 g attached to a string vibrator as illustrated in (Figure). The tension in the string is 90.0 N. When the cord vibrator is turned on, it oscillates with a frequency of lx Hz and produces a sinusoidal moving ridge on the string with an amplitude of 4.00 cm and a constant wave speed. What is the fourth dimension-averaged power supplied to the wave by the string vibrator?

Strategy

The power supplied to the wave should equal the fourth dimension-averaged power of the moving ridge on the cord. We know the mass of the string

$({m}_{s})$

, the length of the string

$({L}_{s})$

, and the tension

$({F}_{T})$

in the cord. The speed of the wave on the string tin be derived from the linear mass density and the tension. The cord oscillates with the same frequency as the cord vibrator, from which we can discover the athwart frequency.

Solution

Brainstorm with the equation of the time-averaged ability of a sinusoidal wave on a string:
$P=\frac{1}{2}\mu {A}^{2}{\omega }^{2}v.$

The amplitude is given, so we need to summate the linear mass density of the string, the angular frequency of the wave on the cord, and the speed of the wave on the string.
We need to calculate the linear density to notice the wave speed:
$\mu =\frac{{m}_{s}}{{L}_{s}}=\frac{0.070\,\text{kg}}{2.00\,\text{m}}=0.035\,\text{kg/m}.$
The moving ridge speed can be found using the linear mass density and the tension of the string:
$v=\sqrt{\frac{{F}_{T}}{\mu }}=\sqrt{\frac{90.00\,\text{N}}{0.035\,\text{kg/m}}}=50.71\,\text{m/s}.$
The athwart frequency can be found from the frequency:
$\omega =2\pi f=2\pi (60\,{\text{s}}^{-1})=376.80\,{\text{s}}^{-1}.$
Calculate the time-averaged ability:
$P=\frac{1}{2}\mu {A}^{2}{\omega }^{2}v=\frac{1}{2}(0.035\frac{\text{kg}}{\text{m}}){(0.040\,\text{m})}^{2}{(376.80\,{\text{s}}^{-1})}^{2}(50.71\frac{\text{m}}{\text{s}})=201.59\,\text{W}.$

Significance

The fourth dimension-averaged power of a sinusoidal moving ridge is proportional to the foursquare of the amplitude of the wave and the square of the athwart frequency of the wave. This is truthful for most mechanical waves. If either the athwart frequency or the amplitude of the wave were doubled, the power would increase by a cistron of four. The time-averaged ability of the wave on a string is also proportional to the speed of the sinusoidal moving ridge on the string. If the speed were doubled, by increasing the tension by a factor of four, the power would too be doubled.

Cheque Your Understanding

Is the time-averaged power of a sinusoidal wave on a string proportional to the linear density of the string?

[reveal-answer q="671846″]Prove Solution[/reveal-answer]
[hidden-respond a="671846″]At starting time glance, the time-averaged ability of a sinusoidal wave on a string may look proportional to the linear density of the string because

$P=\frac{1}{2}\mu {A}^{2}{\omega }^{2}v;$

however, the speed of the moving ridge depends on the linear density. Replacing the moving ridge speed with

$\sqrt{\frac{{F}_{T}}{\mu }}$

shows that the ability is proportional to the square root of tension and proportional to the square root of the linear mass density:

$P=\frac{1}{2}\mu {A}^{2}{\omega }^{2}v=\frac{1}{2}\mu {A}^{2}{\omega }^{2}\sqrt{\frac{{F}_{T}}{\mu }}=\frac{1}{2}{A}^{2}{\omega }^{2}\sqrt{\mu {F}_{T}}.$

[/hidden-answer]

The equations for the energy of the wave and the time-averaged power were derived for a sinusoidal wave on a cord. In general, the energy of a mechanical wave and the ability are proportional to the amplitude squared and to the angular frequency squared (and therefore the frequency squared).

Some other important characteristic of waves is the intensity of the waves. Waves tin likewise be concentrated or spread out. Waves from an earthquake, for example, spread out over a larger area as they motility away from a source, so they do less damage the farther they go from the source. Changing the area the waves embrace has important effects. All these pertinent factors are included in the definition of intensity (I) equally ability per unit expanse:

$I=\frac{P}{A},$

where P is the ability carried by the wave through area A. The definition of intensity is valid for whatever energy in transit, including that carried past waves. The SI unit for intensity is watts per square meter (W/mⁱⁱ). Many waves are spherical waves that move out from a source every bit a sphere. For case, a audio speaker mounted on a mail service to a higher place the ground may produce audio waves that motility away from the source as a spherical wave. Sound waves are discussed in more than detail in the side by side chapter, but in full general, the farther you are from the speaker, the less intense the audio y'all hear. Equally a spherical wave moves out from a source, the surface surface area of the wave increases every bit the radius increases

$(A=4\pi {r}^{2}).$

The intensity for a spherical wave is therefore

$I=\frac{P}{4\pi {r}^{2}}.$

If in that location are no dissipative forces, the free energy volition remain constant as the spherical wave moves away from the source, but the intensity will decrease as the surface surface area increases.

In the case of the two-dimensional circular wave, the wave moves out, increasing the circumference of the moving ridge as the radius of the circle increases. If you toss a pebble in a swimming, the surface ripple moves out every bit a round moving ridge. Equally the ripple moves away from the source, the amplitude decreases. The energy of the moving ridge spreads effectually a larger circumference and the aamplitude decreases proportional to

$\frac{1}{r},$

and not

$\frac{1}{{r}^{2}},$

as in the case of a spherical moving ridge.

Summary

The energy and ability of a wave are proportional to the square of the amplitude of the wave and the square of the angular frequency of the wave.
The time-averaged power of a sinusoidal moving ridge on a cord is plant by
${P}_{\text{ave}}=\frac{1}{2}\mu {A}^{2}{\omega }^{2}v,$

where

$\mu$

is the linear mass density of the string, A is the aamplitude of the moving ridge,

$\omega$

is the angular frequency of the wave, and v is the speed of the moving ridge.
Intensity is defined equally the power divided by the area. In a spherical moving ridge, the expanse is
$A=4\pi {r}^{2}$

and the intensity is

$I=\frac{P}{4\pi {r}^{2}}.$

As the moving ridge moves out from a source, the energy is conserved, but the intensity decreases every bit the area increases.

Conceptual Questions

Consider a cord with under tension with a constant linear mass density. A sinusoidal wave with an angular frequency and aamplitude produced by some external driving force. If the frequency of the driving force is decreased to half of the original frequency, how is the time-averaged power of the moving ridge affected? If the amplitude of the driving strength is decreased by one-half, how is the time-averaged ability affected? Explicate your answer.

[reveal-respond q="fs-id1165038164339″]Bear witness Solution[/reveal-answer]

[hidden-reply a="fs-id1165038164339″]

The time averaged ability is

$P=\frac{{E}_{\lambda }}{T}=\frac{1}{2}\mu {A}^{2}{\omega }^{2}\frac{\lambda }{T}=\frac{1}{2}\mu {A}^{2}{\omega }^{2}v.$

If the frequency or aamplitude is halved, the power decreases by a factor of 4.

[/subconscious-respond]

Circular h2o waves decrease in amplitude as they movement away from where a rock is dropped. Explain why.

In a transverse moving ridge on a cord, the move of the string is perpendicular to the motion of the moving ridge. If this is so, how is possible to movement energy along the length of the string?

[reveal-answer q="806307″]Evidence Solution[/reveal-answer]
[hidden-answer a="806307″]Every bit a portion on the string moves vertically, it exerts a forcefulness on the neighboring portion of the string, doing piece of work on the portion and transferring the energy.[/hidden-answer]

The energy from the sun warms the portion of the earth facing the lord's day during the daylight hours. Why are the N and South Poles cold while the equator is quite warm?

The intensity of a spherical waves decreases as the moving ridge moves away from the source. If the intensity of the wave at the source is

${I}_{0},$

how far from the source will the intensity decrease by a gene of nine?
[reveal-respond q="98685″]Testify Solution[/reveal-answer]
[hidden-answer a="98685″]The intensity of a spherical wave is

$I=\frac{P}{4\pi {r}^{2}},$

if no free energy is dissipated the intensity will subtract by a factor of nine at three meters.[/hidden-respond]

Problems

A string of length 5 m and a mass of 90 g is held under a tension of 100 N. A moving ridge travels down the string that is modeled as

$y(x,t)=0.01\,\text{m}\,\text{sin}(0.40\,{\text{m}}^{-1}x-1170.12\,{\text{s}}^{-1}).$

What is the ability over 1 wavelength?
[reveal-answer q="799885″]Evidence Solution[/reveal-answer]
[hidden-answer a="799885″]

$v=74.54\,\text{m/s,}$

${P}_{\lambda }=91.85\,\text{W}$

[/hidden-respond]

Ultrasound of intensity

$1.50\,×\,{10}^{2}\,{\text{W/m}}^{2}$

is produced by the rectangular head of a medical imaging device measuring 3.00 cm by 5.00 cm. What is its ability output?

The depression-frequency speaker of a stereo set has a surface area of

$A=0.05\,{\text{m}}^{2}$

and produces 1 W of acoustical power. (a) What is the intensity at the speaker? (b) If the speaker projects sound uniformly in all directions, at what distance from the speaker is the intensity

$0.1\,{\text{W/m}}^{2}$

[reveal-respond q="fs-id1165036851502″]Show Solution[/reveal-respond]

[hidden-answer a="fs-id1165036851502″]

$I=20.0\,{\text{W/m}}^{2};$

$\begin{array}{cc} I=\frac{P}{A},\,A=10.0\,{\text{m}}^{2}\hfill \\ A=4\pi {r}^{2},\,r=0.892\,\text{m}\hfill \end{array}$

[/hidden-reply]

To increase the intensity of a wave by a factor of 50, by what gene should the aamplitude exist increased?

A device chosen an insolation meter is used to measure the intensity of sunlight. It has an expanse of

$100\,{\text{cm}}^{2}$

and registers 6.50 Westward. What is the intensity in

${\text{W/m}}^{2}$

[reveal-answer q="fs-id1165037188569″]Testify Solution[/reveal-respond]

[subconscious-answer a="fs-id1165037188569″]

$I=650\,{\text{W/m}}^{2}$

[/hidden-answer]

Energy from the Dominicus arrives at the top of Globe'south atmosphere with an intensity of

$1400\,{\text{W/m}}^{2}$

. How long does information technology take for

$1.80\,×\,{10}^{9}\,\text{J}$

to make it on an area of

$1.00\,{\text{m}}^{2}$

Suppose yous have a device that extracts energy from ocean breakers in directly proportion to their intensity. If the device produces ten.0 kW of ability on a day when the breakers are 1.20 m loftier, how much will it produce when they are 0.600 m loftier?

[reveal-answer q="fs-id1165038204036″]Show Solution[/reveal-respond]

[hidden-answer a="fs-id1165038204036″]

$\begin{array}{cc} P\propto E\propto I\propto {X}^{2}⇒\frac{{P}_{2}}{{P}_{1}}={(\frac{{X}_{2}}{{X}_{1}})}^{2}\hfill \\ {P}_{2}=2.50\,\text{kW}\hfill \end{array}$

[/hidden-answer]

A photovoltaic array of (solar cells) is

$10.0\text{%}$

efficient in gathering solar free energy and converting information technology to electricity. If the average intensity of sunlight on ane day is

$70.00\,{\text{W/m}}^{2}$

, what area should your array accept to assemble energy at the rate of 100 W? (b) What is the maximum cost of the array if it must pay for itself in two years of operation averaging 10.0 hours per day? Presume that it earns money at the charge per unit of 9.00 cents per kilowatt-60 minutes.

A microphone receiving a pure sound tone feeds an oscilloscope, producing a wave on its screen. If the sound intensity is originally

$2.00\,×\,{10}^{-5}\,{\text{W/m}}^{2}$

, just is turned up until the aamplitude increases by

$30.0\text{%}$

, what is the new intensity?

[reveal-answer q="fs-id1165037167410″]Show Solution[/reveal-answer]

[hidden-answer a="fs-id1165037167410″]

$\begin{array}{cc} I\propto {X}^{2}⇒\frac{{I}_{1}}{{I}_{2}}={(\frac{{X}_{1}}{{X}_{2}})}^{2}⇒\hfill \\ {I}_{2}=3.38\,×\,{10}^{-5}\,{\text{W/m}}^{2}\hfill \end{array}$

[/subconscious-answer]

A string with a mass of 0.thirty kg has a length of 4.00 m. If the tension in the string is 50.00 N, and a sinusoidal wave with an amplitude of 2.00 cm is induced on the string, what must the frequency be for an average power of 100.00 W?

The power versus time for a point on a cord

$(\mu =0.05\,\text{kg/m})$

in which a sinusoidal traveling wave is induced is shown in the preceding figure. The moving ridge is modeled with the wave equation

$y(x,t)=A\,\text{sin}(20.93\,{\text{m}}^{-1}x-\omega t)$

. What is the frequency and aamplitude of the moving ridge?

[reveal-answer q="fs-id1165037189407″]Prove Solution[/reveal-respond]

[hidden-answer a="fs-id1165037189407″]

$f=100.00\,\text{Hz},\,A=1.10\,\text{cm}$

[/subconscious-reply]

A string is under tension

${F}_{T1}$

. Energy is transmitted by a wave on the cord at rate

${P}_{1}$

by a wave of frequency

${f}_{1}$

. What is the ratio of the new energy transmission charge per unit

${P}_{2}$

${P}_{1}$

if the tension is doubled?

A 250-Hz tuning fork is struck and the intensity at the source is

${I}_{1}$

at a altitude of one meter from the source. (a) What is the intensity at a altitude of four.00 m from the source? (b) How far from the tuning fork is the intensity a tenth of the intensity at the source?

[reveal-answer q="fs-id1165037208950″]Prove Solution[/reveal-respond]

[subconscious-answer a="fs-id1165037208950″]

${I}_{2}=0.063{I}_{1}$

; b.

$\begin{array}{cc} \hfill {I}_{1}4\pi {r}_{1}^{2}& =\hfill & {I}_{2}4\pi {r}_{2}^{2}\hfill \\ \hfill {r}_{2}& =\hfill & 3.16\,\text{m}\hfill \end{array}$

[/hidden-answer]

A audio speaker is rated at a voltage of

$P=120.00\,\text{V}$

and a current of

$I=10.00\,\text{A}.$

Electrical ability consumption is

$P=IV$

. To test the speaker, a signal of a sine moving ridge is practical to the speaker. Bold that the sound wave moves as a spherical wave and that all of the energy applied to the speaker is converted to audio energy, how far from the speaker is the intensity equal to

$3.82\,{\text{W/m}}^{2}?$

The energy of a ripple on a swimming is proportional to the amplitude squared. If the aamplitude of the ripple is 0.1 cm at a distance from the source of 6.00 meters, what was the amplitude at a distance of 2.00 meters from the source?

[reveal-answer q="fs-id1165036792418″]Show Solution[/reveal-answer]

[hidden-respond a="fs-id1165036792418″]

$2\pi {r}_{1}{A}_{1}^{2}=2\pi {r}_{2}{A}_{2}^{2},\,{A}_{1}={(\frac{{r}_{2}}{{r}_{1}})}^{1\text{/}2}{A}_{1}=0.17\,\text{m}$

[/subconscious-answer]

Glossary

intensity (I): power per unit expanse

parkerbrerefrommen93.blogspot.com

Source: https://opentextbc.ca/universityphysicsv1openstax/chapter/16-4-energy-and-power-of-a-wave/

The ability to do work and what waves transfer

16.4 Energy and Power of a Wave

Learning Objectives

Energy in Waves

Ability in Waves

Example

Power Supplied by a String Vibrator

Strategy

Solution

Significance

Cheque Your Understanding

Summary

Conceptual Questions

Problems

Glossary

0 Response to "The ability to do work and what waves transfer"

Post a Comment

Iklan Atas Artikel

Iklan Tengah Artikel 1

Iklan Tengah Artikel 2

Iklan Bawah Artikel